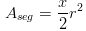Is There a Formula for The 'Perfect' Christmas Tree?

The other week I was waiting for my tram, reading Metro, and I see this article - Treegonometry: Formula for perfect Christmas tree discovered

In other word, a set of equations that will tell you, for example, how much tinsel, or how many baubles are 'required' for the 'perfect' Christmas tree.

Here is the press release, with the actual formulas.

Now, I have a few issues with this. For one thing, it's a bit silly. Surely the perfect tree is a matter of personal preference, and in that sense can't be defined by a set of mathematical rules.

Plus, it seems kind of arrogant to declare that their way is the 'perfect' way, and that (implicitly) any other way is wrong.

Also, the fact that the equations are preposterously simple (perfect thing = constant*tree height) makes me suspicious of them. But, worse than that, it really bothers me that I have no idea how they were derived.

But I'm starting to rant.

The equations in question were actually from a 'study' by the Sheffield University Maths Society (student from my university), and were commissioned by Debenhams (department store). So, I dunno, maybe I'm just bitter that no-one's ever commissioned me to do maths.

Anyway. If they'd said that these were equations for an 'ideal' Christmas tree, then I'd consider that more reasonable. You can say, for example, that the ideal Christmas tree should strike a balance between too many and too few decorations, etc.

So, to that end, I'm going to have a crack at deriving some, more general, 'Equations for an Ideal Christmas Tree' of my own.


Lights

Before we start, there's one simplification we need to make - we will assume that Christmas trees can be approximated to a smooth, regular, right-circular cone - height h, and base radius r.

There's going to be a certain degree of error as a result of this approximation, but I'm not going for perfect.

Okay. So, for lights, there's actually a justifiable basis for an ideal - the ideal tree should have its lights evenly distributed.

Or, to put it another way, you ideally want to wrap your lights such that you have a (roughly) constant light surface density (lights per unit surface area); because you don't want your tree to be covered in clumps and bald spots.

This seems like a vague point; how do you decide on the correct 'density'?

Well, we actually have two constraints to work from: firstly, we have to wrap a single line around our conical shape, and second, the distance (s) between each pair of adjacent lights on a string will be fixed.

From this, we can layout a (hypothetical) grid on the surface of the tree, so that all the lights are equally spaced out.

So, how do we do that?

1) Draw yourself a cone (tree)
2) Draw a grid
3) Draw lights at the points where the grid lines meet
4) Draw in the proper row lines - these represent the actual string of lights

In this cases, I've drawn the grid a little tight, so you'd probably want two strings of lights to get this layout (hence the two colours).

And if you like, you can play with the distances between rows, and the angles, to get some slightly different layouts

The best grid to try for would be a triangular grid, where the distances between each light and the six nearest are the same

So, let's say we've picked a grid. How many light will we need?

There's a nice short-cut to working this out, without having to worry about lengths of spirals on conic surfaces. To do this, we take advantage of the regular grid layout to work out the lights density (lights per unit area).

Each section of grid is roughly a diamond, with all sides about the same length

So with a bit of trigonometry, you can get an approximate equation for the area of this diamond

Then, the density is one over that area (since there's one light per grid diamond).

We then multiply this density by the total conic surface area (excluding the base) to get the total number of lights needed

And, finally, multiplying that by the separation between lights (s), we get the total length needed:

Easy. Though, somewhat more complicated than the L = pi*h equation, derived by SUMS.

So, then, for the triangular grid (theta=60), you get

Cool.

The only problem then is getting the lights to line up on a grid on the actual tree. So... good luck with that.

Realistically, all of this is mostly irrelevant anyway - you can't go out and ask for, say, exactly 5.83m of lights; you buy your lights in pre-cut lengths. Can't get hold of the 'perfect' length of light? Then your tree is imperfect, and you should feel bad.

Anyway. The point is, if you can get your lights more or less even - so that there aren't any clumps or bald spots -, then as far as I'm concerned, you're on to a winner.


Tinsel

What's interesting about their equation for tinsel is this factor of 13/8. Now, again, I don't know how these equations were derived, so I don't know if this was intentional; But, 8 and 13 are consecutive Fibonacci numbers. Why is this important? Well, the Fibonacci sequence is closely related to spirals.

In particular, there's this thing you see in nature; for example, if you look at the spirals on a pineapple, the spirals going in one direction might be 13 and the number in the opposite direction might be 8. Or the numbers might be 21 left and 13 right... The point is, the numbers of spirals on a pineapple are always consecutive Fibonacci numbers (or sometimes Lucas numbers).

And you get the same effect on other things, like the spirals of seeds in the head of a sunflower, or the seeds on a strawberry, or the spirals on a pinecone, or a cauliflower, or all sorts of things. Hell, maybe the branches on a Christmas tree form Fibonacci spirals.

Vi Hart explains it better than me.

So maybe that has something to do with that pre-factor. Or maybe not. It's an interesting tidbit, though.

Anyway.


The thing with tinsel is different people like to do tinsel differently - some like to elegantly drape it across the outer branches, others like to wrap up their tree light they're restraining a hostage. It's a matter of preference. But it's going to affect the amount of tinsel you'll need.

Where tinsel differs from lights is, you're not trying to set up a grid, or get an even surface density. Rather, in this case, you'd probably want to wrap it such that the rows are more horizontal, with a roughly constant vertical separation.

Here's where things get messy; the equation for the length of a spiral on the surface of a cone is given by

I know, right? Maybe you would be better using the SUMS equation for this one.


Extras

The star/angel is going to be some fraction of the height of the tree.

I don't know what the ideal value of the fraction (alpha) would be, but the 10th they came up with seems reasonable.

Baubles, I haven't a clue how they came up with those numbers. The factor of sqrt(17) makes me think some geometry was probably involved, but I dunno.

You would probably want to figure it out as some ideal ornament surface density, Db (like with the lights). In this case, the number of baubles needed would be something like

In fact, if your baubles are all, more or less, the same, you can lay them out on a grid, like the lights. Though, this time, you'd want to have them a little more spaced out, since baubles are much bigger than fairy-lights. But at least this time you don't have the separation constraint.


Thoughts

Here are various things Ben Goldacre, of Bad Science, has said on the subject of commissioned, 'perfect' formulas. Here is an article by mathematician, Simon Singh. Here is an article on BBC News. And here is a collection of such formulas on Apathy Sketchpad.


To be honest, I wouldn't bother with any of this; their equations, or mine. I mean, would you really want a tree that was 'perfectly' decorated? Cold and artificial are the words that come to mind.

And, frankly, I'm not sure my equations would actually work in practice.

I'd say, use your best judgement on how much of everything you'll need, and just do your own thing. Have fun with it!


Our Christmas tree is imperfect. In fact, it's gloriously imperfect. No, seriously, it's a mess.

My ex's family used to construct these massive, elaborate, works-of-art trees; with yearly colour schemes, and matching baubles, and everything. By comparison, she described our tree as kitsch.

But it's adorned with all the baubles, and tinsel, and decorations we accumulated over the last 20-odd years; at least, the ones that haven't been lost or broken. And, in a sentimental sort of way, it is perfect.

Well, okay, not perfect. But, damn it, it's ours.





Oatzy.


[I want you to know, I had no part in decorating that tree.]

[...And, yes, that's a weeping angel on top.]

Picking a Seat on the Tram

When I'm at university, I have to get the tram twice a day. Now I quite like the trams in Sheffield (at least, more than the trains and buses). But if there's anything I can do to get a little more leg room or personal space, then I'm going to try it.

On the way in to university, I get on the tram at the start of the line - i.e. the tram is more or less empty when I get on, so I have pretty much free choice of where I sit. In the main, middle section of the tram, seats are grouped into fours - two forwards, two backwards, face to face - and there are there are 5 rows and 2 columns of these groups.

(The are more seats in the front and rear carriages, but with difference layouts. And I almost never sit in those sections, so I'm ignoring them.)

When the tram's busy, you have to accept that you're probably going to be squashed, and bumping knees with the person across from you, and desperately trying to avoid awkward eye-contact. But when it's less busy, there's the chance for a bit of personal space.

The question is, where's the best place to sit (assuming you have free choice) so you're more likely to get some of that personal space?

Truth be told, I don't actually know. I figured, probably the best place is the forward-facing window seat in either of the 3rd (middle) row groups.

The logic was that a person would either have to sit next to you, or so they're facing backwards - generally less preferable options. And since people are more likely to grab seats nearest doors, the 3rd row is 'best' because it's equidistant from both doors.

Of course, if the front and rear doors aren't used equally, then the choice of row would have to be tweaked.


From that, I started thinking about how people chose which seat to take in a given four-group. And since I have nothing better to do than stare out the window for the 20-odd minute journeys, I figured I'd try to model it.

From empty to full there are 16 possible seat use configurations ('states'). People chose seats based on personal preferences, as well as which seats are already taken. So, for the model, we look at the different states, and consider how they might change with the addition or removal of various numbers of people.

Here's an example, that I sketched, of possible transitions resulting from the addition of one person at a time

The red lines are possible changes of state where the number of people stays the same (usually after the removal of others). More on that later.


Now, the key thing here is that different states, and different transitions are more likely to occur than others.

For eample, a person with free choice is more likely to chose a forward-facing seat (some people tend to feel unwell traveling backwards), and a window-side seat so they've got something to stare at; unless they're planning on making a hasty exit.

So that would theoretically make the backward-facing aisle seat the least popular.

HOWEVER, if someone's already sat in FFWS, then BFAS becomes the more preferable, since it avoids having to sit next to, or directly in front of, some random stranger whose just staring blankly out the window in an unsettling sort of way. Also, personal space.

And from there, if you introduce another person, they're most likely to sit FFAS, since BFWS is a bit awkward to get to and leaves you feeling kind of boxed-in. Plus you have the extra arm room on the aisle-side, and, if necessary, you can turn slightly to give yourself a little more leg room.

And if you add a four person, they have a hell of a time getting to the free seat, but it's their only option. Unless BFAS moves across to the window.

Now, back to those red lines in the diagram. From the 3-config described above, say BFAS leaves. You now have two people sat next to each other. The person in FFAS may then chose to move to BFAS for the sake of more personal space. This is kind of like how physical systems tend towards their lowest energy (least socially awkward) state.


The model itself is actually pretty straight-forward. What you do is construct a stochastic matrix (sometimes called a transition matrix) - basically, a 16x16 'table' that tells you the probability of the seating changing from one state to another. For example

And this works for all possible transitions between all possible states.

The tricky part if determining the probabilities. You could just make random guesses at it. Or, if you were really determined, you could spend a load of time on trams, recording what transitions happen at each stop and how many times they happen. I don't plan on doing either.

But say you have your data and you've constructed your matrix, M. You could do a Monte Carlo type simulation using those probabilities. But with a stochastic matrix you can be more precise.

See, if you calculate M*M, then the entries are the probabilities of moving from state i to j after two 'stops'. And if you calculate M^n, they you get the transition probabilities for after N stops.

And if you add up the entries down each column, j, then you have the probability of being in state j after N stops - that is, you can find the most likely seating configuration after some arbitrary number of stops. You could also use this method to work out the popularity of each seat over all possible states.

Which I think is pretty cool.


And that's all well and good for the groups. But what if you want to model the whole tram? That's where things get tricky, since you have to model how people chose seat groups, which itself depends on what seats are already taken in groups.

Ultimately, it can be modeled the same way, with a transition matrix. Only now, you're working with a 40x40 matrix.

Not difficult, per se, but certainly requires a lot of data and number crunching...

Come to think of it, if you were going to go to the trouble of observing and counting state transitions, you could just count how many times each seat is sat in over some arbitrarily long period to figure out each seat's popularity. Then you just need to try to get the seat next to the least popular.

But counting isn't as fun as modeling.


Oatzy.


[I wonder what normal people think about on public transport..]

Sharing a Burger Between Three

There are several ways to divide a burger evenly between three people.

Probably the best is to cut it radially (like a pizza). It can be tricky working out exactly where to make the cuts, but if you can pull it off, then all the pieces will be roughly identical (topping distribution notwithstanding).

But for the sake of arguing, lets say you want to divide the burger by making two parallel cuts: Where, then, do you make the cuts so that all three people get the same amount of burger?

NB/ This gets quite maths-heavy, so if you're not interested in that sort of thing, feel free to skip right to the end for the solution.



Geometry

For simplicity, we're going to consider the burger as a circle, and make the cuts so that each chunk has the same area. The two cuts are going to be the same distance from, and parallel to, the central axis of the burger, so we only need to consider the position of one of the cuts.

Here's the set-up

We work out the area of the cut-off as the area of the circular segment, minus the area of the triangle.


- Aside: Radians

Radians are basically an alternative way of measuring angles. For maths and physics they're generally more useful than degrees.

They're relatively easy - there are 2pi radians in a full circle, so 2pi radians = 360 degrees

1 radian = 180/pi = 57.3 degrees
1 degree = pi/180 = 0.017 radians, etc.

*    *    *

Back to the circle; with angle x in radians, the area of the circular segment is

The area of a triangle is half base times height..


- Aside: Area of the Triangle

We start by splitting the triangle down the middle, so that we have two identical right angle triangles

The height, l = r*cos(x/2)

The base, b = 2*(r*sin(x/2))

So the area of the triangle is (l*b)/2 = r^2 sin(x/2)cos(x/2)

Finally, use the identity sin(2x) = 2sin(x)cos(x)
to get

*    *    *

So the area of the cut-off is

and it needs to equal a third the area of the circle = 1/3 pi r^2.

So first, we need to find x satisfying

or

Once we have a value for x, we find where to make the cut from

Intermission

The thing about this equation is it doesn't have an exact, analytical solution - to find the solution you have to use numerical methods. Well, I say you have to use numerical methods; these days you can just type the equation into WolframAlpha, and you'll get a solution like *snaps fingers*

Which is nice. I even have the WolframAlpha app on my phone. But when I thought up this question I was on holiday in Sherwood forest, where there was literally no mobile singal.

So that was out of the question. And since I'm not in the habit of carrying a scientific calculator around with me, I was stuck with the basic calculator on my phone. It looks like this:

No trig functions, no square roots, no pi button. It doesn't even do brackets, or have a memory function. Luckily, I am in the habit of carrying around a notepad and pen.

Anyway, there are two ways of working this out with only a basic calculator. The first is 'easier', but only if you know some stuff, and the numbers happen to be nice (in this case, they kind of are). The second is harder, in that it requires more number crunching, but it'll work with any numbers, and can be more precise.

Again, feel free to skip to the solution if you're not interested in the gritty details.



Method One

First of all, here's a graph of the two sides of the equation

hand-drawn with Skitch

We want to find the point at which the two graphs cross. We can see that that happens somewhere between 2pi/3 and pi (120 and 180 degrees). So, lets make a guess that it's exactly halfway between these two values: 5pi/6 (150 degrees).

For the right hand side of the equation: 5pi/6 - 2pi/3 = 0.52

NB/ I'm using pi=3.1416 (rounded to 4 decimal places). If you prefer, you could use the approximation 22/7. The result should be roughly the same.

For the left hand side of the equation, we need to work out sin(5pi/6)

At A-level, we were expected to memorise sin() and cos() of angles 0, 30, 45, 60, 90, and 180 (degrees). We were also expected to know the formulas for sin() and cos() of sums of angles. For sin(), it works like

Why is this important? Well 150 degrees = 180 - 30 (5pi/6 rads = pi - pi/6)

So

And since 0.5 is pretty close to 0.52 - less than 5% error - we can accept the convenience of that answer and say it's close enough.

So our approximate value of x is 5pi/6 = 2.618

[Incidentally, the identity for sin(2x) is just a special case of the above, with a=b=x; i.e. sin(2x) = sin(x+x) = 2sin(x)cos(x)]


Now we just need to work out l/r = cos(x/2) = cos(5pi/12)

For this one, 5pi/12 rads = 75 degrees = 45 + 30, so we can use

So

And we just have to evaluate that. But we don't have a square root button. Now, I just happen to know that sqrt(3) ~ 1.73 and sqrt(2) ~ 1.41.

But I'm just weird like that. Let's say you don't. How do you work it out?


- Aside: Square Roots

There are several ways of working out square roots with just basic operators. For two easy examples:

The first is 'Trial and Improvement' - pick a number, square it, does that give the right answer? If not, pick another number based on whether the last guess was too big or too small.

For example: sqrt(3)

1.5 -> 2.25 -> too small
1.7 -> 2.89 -> too small
1.8 -> 3.24 -> too big
1.75 -> 3.0625 -> too big
1.73 -> 2.9929 -> too small
1.74 -> 3.0276 -> too big
1.735 -> 3.010225 -> too big
1.7325 -> 3.00155625 -> too big
1.732 -> 2.999824 -> too small
etc.

The second method is the "Babylonian Method". It's more systematic, and can converge to the correct answer quicker than guessing. But it can be irritating if your calculator doesn't have a memory function.

It uses the recurrence relation

Basically, you make a guess xn. Divide the number you want to square root (S) by xn. If xn is lower than the actual square root, then S/xn will be greater than it. That means the actual root will be between xn and S/xn, so we make the next guess xn+1 the average of these two values. Repeat until x is sufficiently accurate.

For example: sqrt(2)

x0 = 1.5 -> 2/1.5 = 1.33
x1 = (1.5 + 1.33)/2 = 1.4166.. -> 2/1.4167 = 1.41176..
x2 = (1.4167 + 1.41176..)/2 = 1.41421.. -> 2/1.41421 = 1.41421..

*    *    *

Whatever way you do it, you repeat the process until you get the degree of accuracy you're happy with.

You should get the answer around l/r = 0.259



Method Two

We go back to the equation sin(x) = x - 2pi/3

We still have to find the solution numerically, we still don't have a calculator with a sin() function, and this time the numbers don't work out nicely.

So, the question is, how do we calculate sin(x)?


- Aside: Taylor Expansion

The Taylor Expansion of a function is a way of fitting a polynomial (sums of powers) to a more complicated function. It works like this

Basically, it gives a way of converting a function we can't calculate into an infinite sum of powers of x, which we can calculate.

It's usually expanded around the origin (x0=0), since the equations work out neater. But you can do it around any point, x0=a. This is useful if the value you are trying to calculate is far from x=0. The closer x is to x0=a, the quicker the sum converges.

Even though the expansion is an infinite sum, it's usually sufficient to just take the first few terms, since each additional term makes a smaller and smaller contribution to the sum.

So the trick is working out how many terms you need to include to get some desired level of accuracy.

*    *    *

In this case, I'm going to use the Taylor Expansion of sin(x) around x0=pi, since the approximate value (2.6) is nearer to pi than 0.

Here's what the expansion looks like

So, how many terms do we need to include?

Here's what the graph looks like for different numbers of terms

plotted with WolframAlpha

For a value around 2.6, it can be shown that including the first two terms is correct to ~3 decimal places; the first three terms is correct to ~5 decimal places; the first four terms to ~7 decimal places, etc.

So I would probably go to the third term (for 5dp), but only take the result to 3dp.

NB/ We shouldn't get too hung up on getting an extremely accurate value for x, since we're already getting rounding errors from the factors of pi in the expansion. Also, since we're calculating x to 5dp, we should use pi=3.14159

That means we want to solve

which can't be solved exactly.

So, for finding the correct value (without WolframAlpha), we can use any root-finding method. For what it's worth, I used Trial and Improvement; the other methods are easier with a computer.

But, note that the function is decreasing

So if the guess gives a value greater than zero, you need to increase the value of x (and vice versa).

If you run through all that (I won't go into detail), it gives a value around x=2.605


Alternatively, you could expand around x0=5pi/6 (if you know/can work-out sin and cos of 150 deg without a calculator).

In this case you'd only need up to the term in x^2 (correct to ~4dp). Using this expansion would mean solving a quadratic equation, which is easy. But using this expansion can introduce more rounding errors from the factors of sqrt(3). It's a matter of preference, I guess. The answer should be about the same.


Finally, we need to calculate cos(x/2)

Again, we use the Taylor Expansion to calculate cos(). In this case, we're doing the expansion around x0=0; the expansion is

In this case, you just keep adding terms until the result remains approximately constant to some desired degree of accuracy (3pd).

This gives a value around l/r = 0.265



So What is the Real Answer?

Once I got to somewhere where I could get at WolframAlpha, I checked the real numbers; here are the results:

The approximation of x from Method One (2.618) is an over estimate by ~0.5%, which is relatively acceptable. The approximation from Method Two (2.605) is correct to 3 decimal places, which is definitely acceptable.

And for the value of l/r

From Method One (0.259), the approximation is an under estimate by 2%, and correct to 2 decimal places, so is probably acceptable. The approximation from Method Two (0.265) is, again, correct to 3 decimal places. So that is also acceptable.

So, if the numbers happen to be convenient and you know some trigonometry, you're probably as well using Method One. If not, or if you just want more accuracy, then go for Method Two.



Applying the Results

The results are actually quite nice, in terms of practical application (dividing up a burger). The ratio of the radius (0.265) being close to one quarter, you find the cuts like this

That is, find the central axis, then find the (imaginary) line halfway between the centre and the edge - make the cut halfway between the centre and this imaginary line (maybe cut an extra hair's breadth towards the edge). Repeat on the other side.

Easy.


So, now you know. Obviously, all this applies to dividing any circular thing evenly between three people. You could probably even adapt the methods for sharing between even more people.

And in theory, You could do all this with just pen and paper (no calculator). Though you probably wouldn't want to. I know I wouldn't..


Oatzy.


[Wow, I really managed to stretch that one out.]

Too Many Lotteries

So recently I'd gotten really into this TV show - it's called 'Life', and it's a 'murder of the week' crime drama starring Damian Lewis. Lewis plays Detective Crews, who was previously in prison for a muder he didn't commit, and he's into zen and such. It's a bit silly. But in an endearing sort of way.

Unfortunately, the only way I could watch it (short of buying the DVDs) is to stay up til 4am. Which isn't really a problem; I don't have anything to get up for. Though it is tricky getting to sleep as the sun's coming up.

Anyway, the point is, by 4am, there are only, like, four adverts on rotation (on FX, at least). These are: upcoming shows on FX, whatever product JML is flogging, that tacky accident helpline ad with Esther Rantzen, and this 'Win Trillions' thing.

Win Trillions is a lottery sindicate thing - from what I understand, it works like this: a bunch of people from around the world get together, each buying tickets for their respective countries' lotteries. If one of the sindicate members wins, they split the winnings with their fellow sindicate members (and presumedly, Win Trillions takes a cut).

Really, though, the details aren't that important. What is important is their slogan - "play more lotteries, get more chances".

Which isn't a false claim. It's just kind of misleading; playinng more lotteries does mean more chances of winning, but only in a similar way as buying more tickets for a single lottery means more chances of winning - your odds of winning are so small to begin with (~1 in 13,983,816) that you'd have to play thousands of lotteries (or buy thousands of tickets) to significantly improve your chances. And there are only so many lotteries in the world.


Strategies

You'll notice I used the word 'similar' back there; the fact is, buying more tickets, and playing more lotteries don't 'improve' odds in the same way.

So the question is, which is the better strategy - buying many tickets for a single lottery, or buying one ticket for each of several lotteries?

First, imagine I have a dice; I'm going to roll the dice, and you have to bet on the outcome. Your choice is this - would you rather place three bets on one dice roll, or one bet on each of three dice rolls?

For the first case, say you place your bets on it being 1, or 2, or 3. Your odds of guessing the correct number (and winning) is 3 in 6 (=0.5).

For the second case, say you bet that each of the rolls will be 6. Then your odds of winning 'something' is odds of winning just one of the dice rolls, plus the odds of winning just two of the dice rolls, plus the odds of winning all three.

Which is trickier to work out. For example, the odds of winning the first roll only, is odds of getting the first roll right (1 in 6), and not getting the second roll right (5 in 6) and not getting the third roll right (5 in 6) - which makes the probability of getting just the first roll right = 1/6 * 5/6 * 5/6 = 25 in 216

And you have to work out the probabilities for all possible winning outcomes (in this case, there are seven winning outcomes, one losing.).

Alternatively, we can use the trick that the odds of winning something is one  minus the odds of winning nothing. The odds of winning nothing is the odds of getting all the bets wrong: 5/6 * 5/6 * 5/6 = 125 in 216 (0.58)

So the odds of winning something is 91 in 216 (=0.42)

So for this dice game, it's better to place your three bets on a single dice roll.

But what about in general?

We say each game has a probability p of winning, and that we're going to place n bets (with n>1).

For the single game bet, the probability of winning is n*p

For the multi-game bet, the probability of winning is

Which is bigger?

Well for n = 1, the games are idential. For n = 2, the single game is 2p, the multi-game is 2p - p^2; the single game has higher odds. For n >= 1/p, the single game gives a probability greater than or equal to 1 - i.e. guaranteed win - where the multi-game always gives a values less than one; again, the single game gives better odds.

So it's looking like the single game is always better. But how do we prove it?

For this, we look at the binomial expansion of (1-p)^n

A Binomial Expansion works like this

where k! is the factorial of k. For example: 2! = 2*1; 3! = 3*2*1; 4! = 4*3*2*1; etc.

In this case, x = 1 and y = -p, so the expansion becomes

Which makes the probability of winning the multi-game

So whether or not the mutli-game has higher odds than the single game depends on the sum of the terms after np.


First, we compare the k-th and (k+1)-th (adjacent) terms in the expansion

Which simplifies to

The righthand side has it's maximum value when n is maximum. We previously defined the maximum value of n as 1/p, so

since p and k are always greater than 0.

Which means

In other words, each term in the expansion is smaller than the ones before it.

This means that, if we pair up terms,

i.e. the sum of each pair will be negative. Therefore, the sum of all the pairs (all the terms in the expansion after np) is negative. So necessarily

Which means the odds of winning the single game is ALWAYS better than the odds of winning the multi-game.


Pay-Outs

You might have noticed that the single game has a single possible payout, where playing the multi-game means you could win double, triple, or even more of the prize. So the odds of winning the multi-game are lower, but you stand to win more.

Does that mean it's worth the extra risk?

For this, we look at the expected winnings for each game. The expected winnings is calculated as the probability of winning multiplied by the prize amount.

So if you were guessing the outcome of a dice roll, and the prize was £1, then the expected winnings from playing that game would be ~17p.

Or think of it like this - if you had to guess the outcome of a dice roll six times, you would expect to be right once out of six. So your expected prize for 6 rolls is £1, or 17p per roll.

For this single game, the payout is easy: w*n*p (where w is the prize for winning one game)

For the multi-game it's more complicate. We can't use the same trick as last time; instead, we have to work out odds of winning one game times prize from one game + odds of winning two games times prize from two game + etc.


The general form is the series sum

Which can be manipulated and simplified to give

Now, if we expand it out again

Then there's a factor of np in each term, so we can take that outside the bracket

And since n is some arbitrary interger, we can substitute m = n - 1 and put it back in summation form

Now, you might notice the summation part is actually a binomial expansion; in this case we have x = (1 - p) and y = p, so

For all values of p and m = n - 1.

Therefore, the expected payout of the multi-game is always w*n*p


You'll notice that this is the exact same expected payout as for the single game. As in, regardless of which strategy you use, your expected payout is the same.

So the question is, would you rather a low risk low prize game, or a high risk (potentially) high prize game?


Complexities

You'll notice for this I assumed all lotteries have the same odds of winning and the same prize amount. This is not necessarily true.

Even so, from this, the best strategy would be to find the lottery with the best single game single bet expected payout (prize * probability of winning), and buy several tickets for that game, rather than spread your money around.

The other thing in lotteries is you can usually win smaller prizes for matching fewer numbers. But I don't imagine that changes which strategy is best.

So now you know. For what it's worth. The odds of winning playing, say, 70 tickets on one lottery is only about two 10,000ths of a percent better than playing one ticket on 70 lotteries.


Incidentally, those derivations up there count as mathematical proofs. So these conclusions I've drawn are irrefutable*. And the results apply to any game where you're placing bets on a single probability random outcome - not just lotteries.


Oatzy.


[*- Assuming I haven't cocked it up...]